On a related note, I found out today that the Tufts Department of Mathematics is starting a Math Circle. I will have to check it out!
But in Melrose, we finished today’s session of Math Circle playing the Keypad Game, which I first read about on page 1 in Gilman’s Book. As the earlier games we played were not numerical, I wanted to get into a game with some arithmetic.
The full details of the Keypad Game can be read about in a 2007 edition of the College Mathematics Journal. They have an article called the Number-Pad Game by Alex Fink and Richard Guy. This is a fun game which can be played on a normal telephone key pad.
You can include the zero button, but for the game we played in class, we did not use the zero. The rules are simple. Firstus pushes any button. Secondus must push a button in the same row or column. Then firstus pushes another button in the same row or column as secondus (and may push the original button). This goes back and forth until one player gets MORE than 30. That player loses.
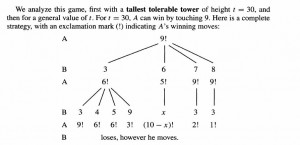
You can choose any # to be higher than, but we started out with the number 30 for a trial. After the students played each other, I challenged them to beat me, which none of them could, but I cheated. I knew that if I started playing with the # 9, and followed a certain pattern, it was impossible for them to beat me.
There is an interesting choices for the #30 as follows.
How to win for the number 30 starting with the number 9
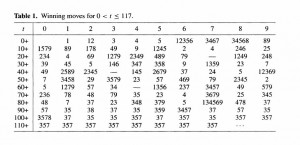
As Fink notes, this game can be played for any number, or as he calls it, the Tallest Tower. If you look in the table, you see that for the # 30, both 3 and 9 are winning first moves, but 9 will get you there quicker.
How to win for any number
Have a good February Vacation!
